Vous trouverez ci-dessous le planning du séminaire d’Analyse
Fonctionnelle pour l’année 2018-2019. Le programme de l’année en cours se trouve
ici.
Pour contacter la responsable (Yulia Kuznetsova) : yulia.kuznetsova univ-fcomte.fr.
Pour s’abonner au séminaire : ACM.
Juin
Embeddings of uniform Roe algebras
Given a metric space $X$, the uniform Roe algebra of $X$ is denoted by $^*_u(X)$. Many authors have studied rigidity questions related to isomorphisms between uniform Roe algebras. In this work, we study embeddings of uniform Roe algebras and what are the geometric properties of the metric spaces which are stable under such embeddings (this is a joint work with Ilijas Farah and Alessandro Vignati).
-Mardi 25 juin: Tiju Cherian John, Indian Statistical Institute
Mai
Journées Besançon-Neuchâtel
Programme : https://lmb.univ-fcomte.fr/Journees-Besancon-Neuchatel-2019
-Mardi 14 mai: J.P. McCarthy, Cork.
Some unresolved and unexplored aspects of Random Walks on Quantum Groups
We briefly present the recent history of the use of the Diaconis\T1\textemdash Shahshahani Upper Bound Lemma in the study of random walks on quantum groups, including in a series of papers by Amaury Freslon. At this point we pause and have a look at the study of random walks on classical groups, and point out a number of aspects, some fundamental, of that theory that are unresolved and/or unexplored in the quantum setting. This talk does not present new results, and comprises a short survey followed by a presentation of problems potentially ripe for an attack.
-Mardi 7 mai: Claus Köstler, Cork.
Distributional Symmetries in Noncommutative Probability
This is an introductory lecture to the mini-course given during 4 further sessions.
Avril
Sur la géométrie métrique des graphes entrelacés de Kalton
Une approche à la fois naturelle et efficace pour classifier des objets mathématiques d\T1\textquoteright un certaine catégorie est la découverte de propriétés invariantes par isomorphisme de cette catégorie. En géométrie métrique, nous nous intéressons à des propriétés stables par certains types de plongement (Lipschitz, uniforme, grossier, etc). Nous recherchons souvent ces invariants sous la forme d\T1\textquoteright inégalités de concentration pour des fonctions Lipschitziennes à valeurs dans un espace de Banach. En effet, dans un papier fondamental dans cette théorie, Kalton définit un invariant grossier sous la forme d'un phénomène de concentration pour les fonctions Lipschitziennes définies sur une famille particulière de graphes métriques. Il s'agit de la propriété (Q) et des graphes entrelacés de Kalton. Dans cet exposé, nous mettrons en évidence un invariant grossier assez proche mais différent de celui de la propriété (Q). Il consiste dans le fait de ne pas contenir grossièrement la famille des graphes de Kalton sans pour autant vérifier l'inégalité de concentration mentionnée ci-dessus. Ce travail est basé sur une compréhension plus profonde de la géométrie des graphes entrelacés et de la structure asymptotique de certains espaces de Banach. Nous terminerons l\T1\textquoteright exposé par l\T1\textquoteright étude d\T1\textquoteright une propriété plus faible consistant aussi en un phénomène de concentration des graphes entrelacés.
-Mardi 9 avril: Gilles Godefroy, IMJ.
Séminaire d'Analyse Fonctionnelle
Mars
Duality for Hopf holomorphically finitely generated algebras
Inspired by the duality theory of locally compact quantum groups, we discuss a class of topological Hopf algebras that can be considered as algebras of 'holomorphic functions on quantum complex Lie groups'. A Hopf holomorphically finitely generated (HFG) algebra is introduced as a topological Hopfalgebra that is a quotient of Taylor's algebra of free entire functions. For every Hopf HFG algebra $H$, the dual topological Hopf algebra $H^\circ $ can be defined. We talk over conditions under which $H^\circ $ is HFG. The natural commutative example of a Hopf HFG algebra is $\mathcal O(G)$, the algebra of holomorphic functions on a complex Lie group $G$. It is shown, under the assumption that $G$ is connected, that $\mathcal O(G)^{\circ \circ }\cong \mathcal O(G)$ iff $G$ is linear, i.e., admits a faithful finite-dimensional holomorphic representation.
-Jeudi 7 mars à 13h30: Oleg Aristov, Moscow.
Commutative and noncommutative $C^\infty $-functional calculus
In Noncommutative Differential Geometry, some dense subalgebras of $C^*$-algebras are considered. But there is still no an axiomatic definition of 'function algebras' on noncommutative $C^\infty $-manifolds or, more generally, $C^\infty $-spaces. One of main requirement to a candidate for the title is stability under $C^\infty $ -functional calculus for several commuting self-adjoint elements. We exhibit conditions that guarantee existence of a $C^\infty $-functional calculus on the joint spectrum of a commuting tuple of self-adjoint elements on a $C^*$-spectral Arens-Michael $*$-algebra and compare them to the Kissin-Shulman differential norm condition. We also discuss how to construct an enveloping functor that maps algebras of polynomials to algebras of $C^\infty $-functions and that is compatible with Pontryagin duality for abelian real Lie groups. The more distant goal is to extend Pontryagin duality from abelian Lie groups to all Lie groups.
-Mardi 12 mars: Uwe Franz, LMB.
Monotone Increment Processes, Classical Markov Processes and Loewner Chains
We prove a one-to-one correspondence between certain decreasing Loewner chains in the upper half-plane, a special class of real-valued Markov processes, and quantum stochastic processes with monotonically independent additive increments. This leads us to a detailed investigation of probability measures on the real line with univalent Cauchy transform. We discuss several subclasses of such measures and obtain characterizations in terms of analytic and geometric properties of the corresponding Cauchy transforms. Joint work with Takahiro Hasebe and Sebastian Schleissinger.
-Mardi 19 mars: Emiel Lorist, Delft.
Singular stochastic integral operators
Singular stochastic integrals of the form$$ S_K G(t) :=\int_0^\infty K(t,s) G(s) ,\mathrm d W_H(s), \qquad t\in \mathbb R_+,$$appear naturally in questions related to stochastic maximal regularity. Here $G$ is an adapted process, $W_H$ is a cylindrical Brownian motion and $K$ is allowed be singular.In this talk I will introduce Calder\'on--Zygmund theory for such singular stochastic integrals with operator-valued kernel $K$.I will first discuss $L^p$-extrapolation under a H\"ormander condition on the kernel. Afterwards I will treat sparse domination and sharp weighted bounds under a Dini condition on the kernel, leading to a stochastic analog of the solution to the $A_2$-conjecture. The developed theory implies $p$-independence and weighted bounds for stochastic maximal $L^p$-regularity both in the complex and real interpolation scale. This leads to mixed $L^p(L^q)$-theory for several stochastic partial differential equations, of which I will give a few examples. This talk is based on joint work with Mark Veraar.
-Mercredi 20 mars à 16:30: Frédéric Patras, Nice.
Jeudi 21 - Vendredi 22 mars: Julien Bichon, Malte Gerhold Anna Kula, Martin Lindsay, Michael Schuermann, Adam Skalski, Moritz Weber
Journées Thématiques : Cohomology of Compact Quantum Groups and Related Topics
See the dedicated web-page
Février
Extremal structure in Lipschitz-free spaces
We will review the current knowledge on extremal structure of the unit balls of Lipschitz-free spaces. We will focus on the characterization of various types of extremal molecules, and highlight how they establish a duality between linear alignment on the free space and metric alignment on the underlying metric space.
-Mardi 12 février à 14h45: Noé de Rancourt, .
Séminaire d'Analyse Fonctionnelle
-Mardi 5 février à 14h45: Yuki Ueda, .
On free multiplicative convolution with the free normal distributions on the unit circle
The free multiplicative convolution means a distribution of product of freely independent random variables. In general, it is very difficult to find a density function of free multiplicative convolution of two probability measures on the unit circle. However, Zhong found a density formula of free multiplicative convolution with the free normal distributions on the unit circle. We will talk about unimodality for free multiplicative convolution with the free normal distributions on the unit circle by using Zhong's density formula. This is a jointwork with Takahiro Hasebe in Hokkaido university.
Janvier
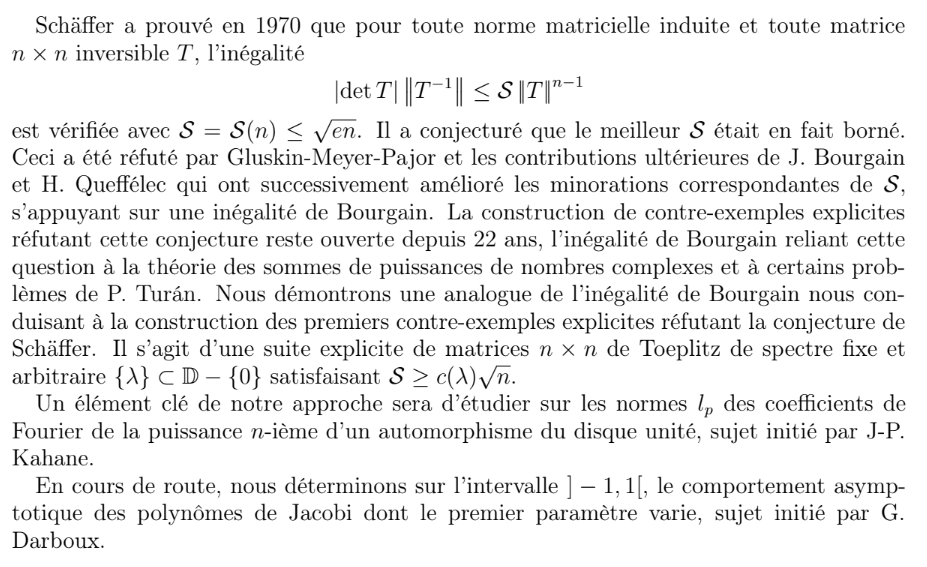
-Mardi 8 janvier : Rachid Zarouf, Institut de Mathématiques de Marseille
Contre-exemples explicites réfutant la conjecture de Schäffer
-Mardi 15 janvier : Gonzalo Flores, Universidad de Chile
Linear structure of functions with maximal Clarke subdifferential
We prove that the set of real valued Lipschitz functions defined over finite dimensional spaces whose Clarke subdifferential is maximal at every point contains a linear subspace of uncountable dimension. This result goes in the line of a previous result by J. Borwein and X. Wang that shows some type of density in a similar context. Nevertheless, contrary to that result, our aproach is constructive. Moreover, in our setting we establish the spaceability of this property in the set of Lipschitz continuous functions. Joint work with A. Daniilidis.
-Mardi 22 janvier : relâche (école d'hiver)





